Since the end of the 20th century, quantum theory has experienced a revival which has resulted in the emergence of quantum technologies using all the power of quantum interference to measure, simulate, communicate and calculate. At the same time, this «second quantum revolution» challenges our understanding of quantum theory and pushes us to go beyond the purely utilitarian vision of the Copenhagen interpretation.
The objective of this lectures is to present the deep relationships between quantum mechanics, information theory and computation. These relationships emerged over time after Shannon's work on information theory, work on physical limits to computation studied by Landauer and Bennett in the 1980s and finally, since the 1990s, the development of quantum information both in physics and computer science and also at both the theoretical and experimental levels.
The aim is to show students how this new understanding of the quantum world has been nourished by contributions from several disciplines (physics and computer science) but also by technological progress as well as by fundamental and more applied questions. Thus, throughout the course, the concepts will be discussed on examples of real physical systems and through quantum physics experiments carried out with them in order to illustrate Landauer's idea: «Information is physical».
Synopsis
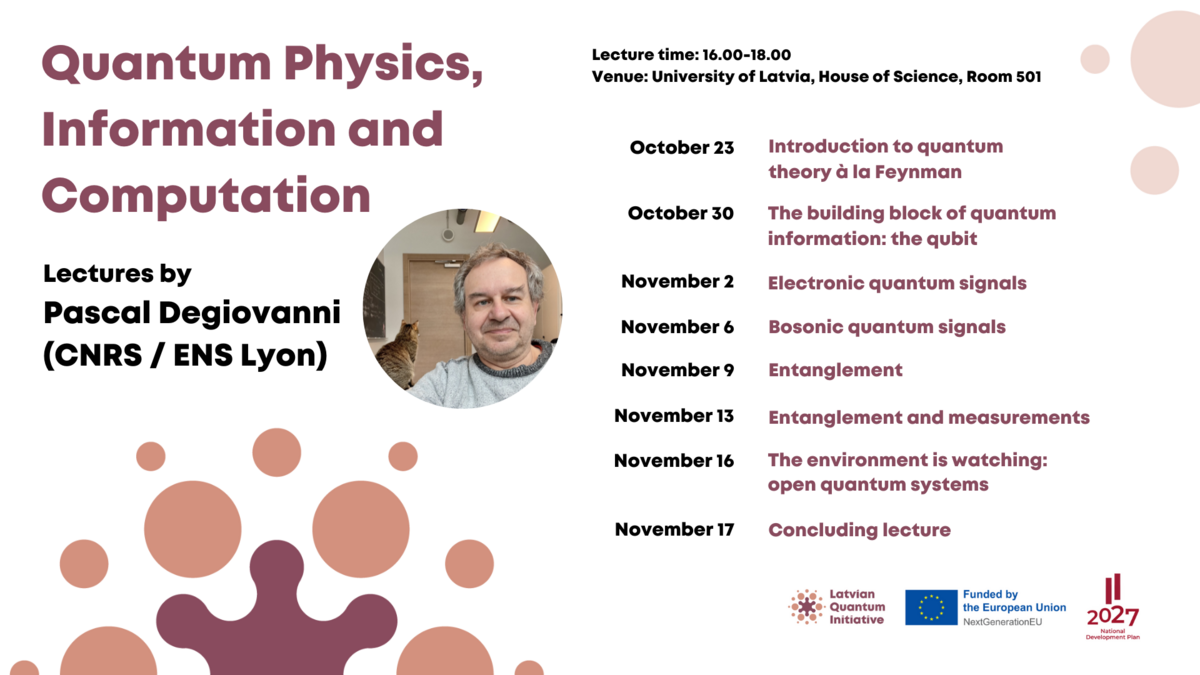
Introduction to quantum theory à la Feynman | October 23
- Path integral formulation of quantum mechanics and its relation to the usual formulation.
- Topological phase factors: Aharonov-Bohm effect, quantum statistics (fermions, bosons and anyons)
The building block of quantum information | October 30
- The Poincaré-Bloch sphere
- Examples of physical realizations of qubits
- The Bennett-Brassard quantum cryptography protocol (BB84)
- Estimating the quantum state of a qubit: Massard and Popescu theorem for N=1 and large N realizations of the system.
Electronic quantum signals | November 2
- The integer quantum Hall edge channel
- Electronic coherence (first and second order) and their relation to observable quantities
- Electronic interferometry as quantum signal processing
- Electronic atoms of signal
Bosonic quantum signals | November 6
- The basics: space of states
- Coherent states
- The quantum oscillator in phase space: the Wigner function
- Quantum state reconstruction in cavity and circuit QED
- The quantum transmission line
- Classical radiation and coherent states
- Glauber coherences
- Photonic atoms of signal
Entanglement | November 9
- States of a composed quantum system, relative states, statistical ensemble and reduced density operators.
- Schmidt decomposition of an entangled pure state, einvariance, purifications and the Gisin Hughston, Josza and Wooters theorem.
- Why is it that quantum correlations stronger than classical ones?
Entanglement and measurements | November 13
- Generalized measurements from entanglement
- Weak vs strong measurements
- The quantum limit for weak measurements
The environment is watching: open quantum systems | November 16
- Quantum decoherence
- Quantum trajectories
- Markovian dynamics: Lindblad equations and their unraveling by quantum trajectories
- Example: monitoring a qubit via it’s emitted radiation
Concluding lecture | November 17
- will depend on the coverages of the previous topics
Lecture time: 16.00-18.00
Venue: University of Latvia, House of Science, Room 501 (5th floor)